Applicative programming in Ruby: advanced behaviors

Part 1 | Part 2

In the previous post we implemented safe currying on applicative functors. We used a container called Either to create these interfaces. In this post we will go even further and create applicative lists and parsers.

Applicative array

Let’s take a look how functors can be implemented for lists:

class ApplicativeArray < Array
  include Functor
  def fmap(&fn) = map(&fn.curry)

  include Applicative
  class << self
    def pure(x) = ApplicativeArray.new([x])
  end

  def ^(other)
    ApplicativeArray.new(flat_map { |fn| other.fmap(&fn) })
  end
end

Functor#fmap is almost a regular map, but it uses curry call functions inside the list partially. pure takes the value and wraps it into the array. Application (^) takes function from another array and applies it to each element of the current array. Let’s try it:

def plus(x, y) = x + y
def mult(x, y) = x * y

array_with_functions = ApplicativeArray.new([method(:plus), method(:mult)])
array_with_args = ApplicativeArray.new([2, 7])
array_with_args_2 = ApplicativeArray.new([3, 5])

array_with_functions ^ array_with_args ^ array_with_args_2
# => [5, 7, 10, 12, 6, 10, 21, 35]

Why 8 elements?

1. plus is applied to two elements;
2. mult is applied to same elements, getting 4 partially applied functions;
3. these functions are applied to each of two elements getting 8 elements in total.

Can we apply functions to the corresponding element only? Sure, in Haskell this type of the list is called ZipList:

class Applicative::ZipList
  attr_reader :list

  def initialize(list) = @list = list

  include Applicative::Functor
  def fmap(&fn) = @list.map(&fn.curry)

  include Applicative::ApplicativeFunctor

  class << self
    def pure(x) = Applicative::ZipList.new(repeatedly { x })

    private

    def repeatedly(&block)
      Enumerator.new do |y|
        loop { y << block.call }
      end.lazy
    end
  end

  def ^(other)
    eager_list =
      if @list.is_a?(Enumerator::Lazy) && other.list.is_a?(Enumerator::Lazy)
        @list
      else
        @list.take(other.list.length)
      end

    Applicative::ZipList.new(eager_list.zip(other.list).map { |fn, x| fn.curry.(x) })
  end
end

Implementation is slightly more complex, because we have to handle endless lists: we need to do that because pure returns it. Here is an example:

plus = lambda { |x, y| x + y }
mult = lambda { |x, y| x * y }

functions = ZipList.new([plus, mult])
args = ZipList.new([2, 7])
args_2 = ZipList.new([3, 5])

(functions ^ args ^ args_2).list # => [5, 35]

Let’s try the same thing but using pure, that constructs an endless list for us:

functions = ZipList::pure(plus) # endless
args = ZipList::pure(2) # endless
args_2 = ZipList.new([4, 6, 8]) # finite

(functions ^ args ^ args_2).list.eager.to_a # => [6, 8, 10]

In this case we get only 3 elements (2 + 4, 2 + 6 and 2 + 8).

Applicative parser

Let’s take a look at more complex application of applicatives: an applicative parser. We start with the new data structure called Pair, which can hold two values:

class Pair
  attr_reader :fst, :snd

  def initialize(fst, snd)
    @fst = fst
    @snd = snd
  end

  def deconstruct = [@fst, @snd]

  include Applicative::Functor
  def fmap(&fn) = Pair.new(fst, fn.(snd))
end

It will be a functor, where fmap applies function to the second element of the pair, without changing the first one. Now let’s define a class that presents a generic parser:

class Parser
  def initialize(&parse_fn) = @parse_fn = parse_fn

  def parse(s) = @parse_fn.(s)
end

parse_fn function should accept and parses some string. When parsing fails it should return Left with error message, otherwise — Right with the Pair. The first element of the Pair is the remaining string, the second element is the thing we just parsed. For instance, here is a parser that looks for a character 'x' in the beginning of the string:

x_parser = Parser.new do |s|
  if s.empty?
    Left("unexpected end of input") # because there is no 'x' at the beginning of the empty string
  else
    char = s[0]
    char == 'x' ? Right(Pair.new(s[1..], char)) : Left("unexpected #{char}")
  end
end

x_parser.parse("") # => Left("unexpected end of input")
x_parser.parse("ab") # => Left("unexpected a")
x_parser.parse("xab") # => Right("ab", "x")

Let’s implement Functor for the parser. fmap accepts some function that can be applied to the result of the parser and constructs a new parser. Note that we do not parse anything, just build a new parser:

class Parser
  include Applicative::Functor

  def fmap(&fn)
    Parser.new do |s|
      parse(s).fmap { |pair| pair.fmap(&fn) }
    end
  end
end

x_parser.fmap(&:upcase).parse("xab") # => Right("ab", "X")

Let’s add a couple of helpers to build parsers easily:

class Parser
  class << self
    def satisfy(&predicate)
      Parser.new do |s|
        if s.empty?
          Left("unexpected end of input")
        else
          char = s[0]
          predicate.(char) ? Right(Pair.new(s[1..], char)) : Left("unexpected #{char}")
        end
      end
    end

    def char(c)
      satisfy { |current| c == current }
    end

    def string(str)
      Parser.new do |s|
        if s.start_with?(str)
          Right(Pair.new(s[str.length..], str))
        else
          Left("unexpected #{s}, expected #{str}")
        end
      end
    end
  end
end

Now let’s make our parser Applicative to make it possible to combine parsers:

class Parser
  include Applicative::ApplicativeFunctor

  class << self
    def pure(value)
      Parser.new { |s| Right(Pair.new(s, value)) }
    end
  end

  def ^(other)
    Parser.new do |s|
      case parse(s)
      in Applicative::Either::Right(Pair(s1, g))
        case other.parse(s1)
        in Applicative::Either::Right(Pair(s2, a)) then Right(Pair.new(s2, g.(a)))
        in left then left
        end
      in left then left
      end
    end
  end
end

pure creates a new parser that always “parses” any value we pass, returning the remaining string as is. Application creates a new parser using following steps:

• if current parser cannot parse a string — we return Left;
• otherwise it returns us the remaining string s1 and the result a, we tries to parse s1 with the second parser:
  • if second parser fails — we return Left;
  • otherwise second pair returns us a function g (because we’re in the Applicative), so we return Right:
    • the first element is a string that remained from the second parser;
    • the second element is a result of calling g with a.

This is how it works:

parser = (
  Parser.pure(lambda { |a, b| a + b }.curry) ^
    Parser.char('A') ^
    Parser.string("BC")
)

parser.parse("ABC") # => Right ("", "ABC")

As you see, ^ operates as the AND operator: in this example we ask parser to find us character A followed by string BC. What about OR?

module Alternative
  def |(other) = raise NotImplementedError
end

class Parser
  include Alternative

  def |(other)
    Parser.new do |s|
      case parse(s)
      in Applicative::Either::Right(r) then Right(r)
      in _
        case other.parse(s)
        in Applicative::Either::Right(r) then Right(r)
        in left then left
        end
      end
    end
  end
end

The new method | tries to parse with the first parser, if it fails — with the second, and returns the result.

Let’s combine everything together:

parser = (
  Parser.pure(lambda { |a, b, c| a + b + c }.curry) ^
    (Parser.char('A') | Parser.char('B')) ^ # we want A or B ...
    Parser.char('C') ^ # ... then C ...
    Parser.string("42") # ... then 42
).fmap(&:downcase) # parser is functor, so `fmap` can be used to transform the result

parser.parse("AC42D") # => #<Either::Right:… @value=#<Pair:… @fst="D", @snd="ac">>
parser.parse("BC42D") # => #<Either::Right:… @value=#<Pair:… @fst="D", @snd="bc">>
parser.parse("DCB") # => #<Either::Left:… @error="unexpected D">

Impressive, right?

Traversable

Let’s try to build something on top of applicatives.

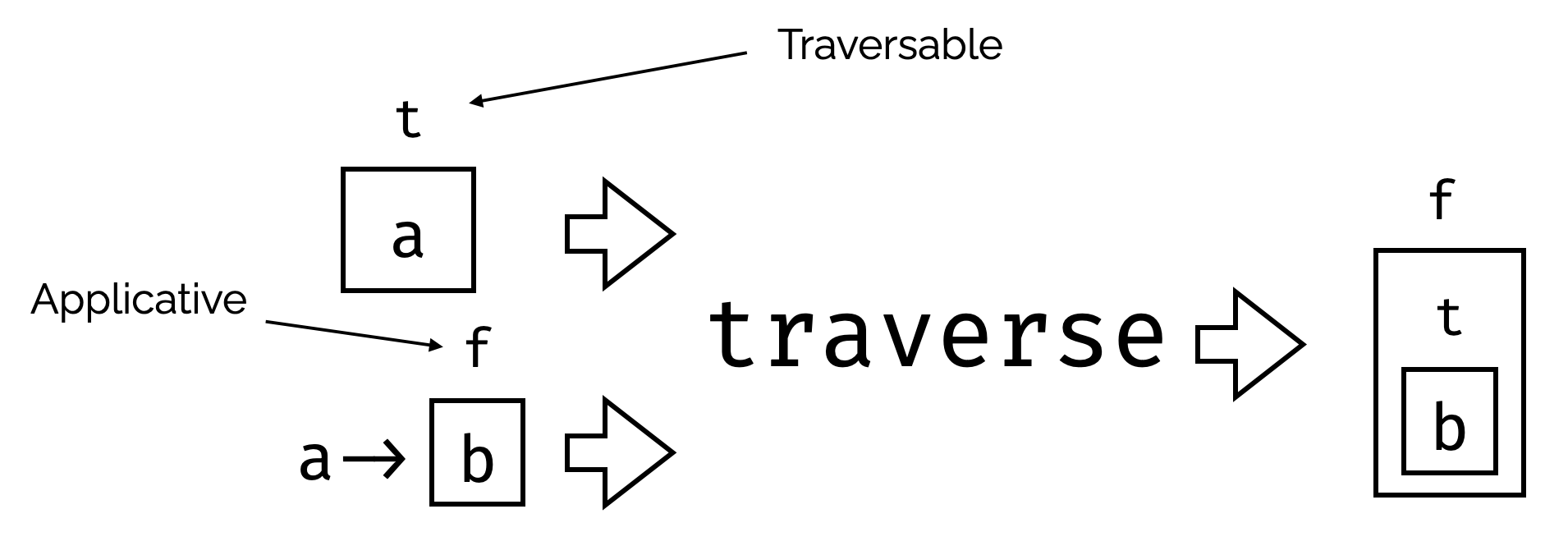
Imagine that you have some applicative container f which has a function from a to b inside. For instance, this function accepts Either Int and tries to increment it returning Either Int:

increment = lambda { |either_value| either_value.fmap { |value| value + 1 } }

increment.(Right(42)) # => Right(43)
increment.(Left("error")) # => Left("error")

Then, we have a new module called Traversable. It has a single method called traverse:

module Traversable
  def traverse(applicative_class, &_fn) =
    raise NotImplementedError
end

This function accepts some applicative_class (which includes Applicative) and a function we described above. The result of the call should be the instance of the same Applicative that contains the instance of the the same Traversable. Traversable should contain the value, that was returned by fn applied to the data inside the initial traversable.

That sounds smart, so let’s just write it and run. Here is the implementation for array:

class ApplicativeArray < Array
  include Traversable

  def traverse(applicative_class, &fn)
    return applicative_class::pure([]) if empty?

    x, *xs = self

    applicative_class::pure(lambda { |ta, rest| [ta] + rest }) ^
      fn.(x) ^
      ApplicativeArray.new(xs).traverse(applicative_class, &fn)
  end
end

And the example of usage:

increment = lambda { |maybe_value| maybe_value.fmap { |value| value + 1 } }

ApplicativeArray.new([Right(1), Right(3), Right(5)])
  .traverse(Either, &increment) # => #<Either::Right:... @value=[2, 4, 6]>

ApplicativeArray.new([Right(1), Left("error")])
  .traverse(Either, &increment) # => #<Either::Left:... @error=“error">

Now we can describe the behavior in the easier way: traverse goes through some data structure containing values in containers, applies the function to the value in container and extracts container to the top. In our example, list of Either Int becomes Either list of Int:

• if initial list has only Right then Right will be outside;
• if there are any lefts — the first one will be extracted.

Outro

In this post we implemented two different applicative functors for lists, created a simple (but yet powerful!) applicative parser and made our lists traversable. As you see, applicative functor is a powerful abstraction that helps to describe complex behavior and hide it inside the container.

Hopefully one day we will make something out of it!